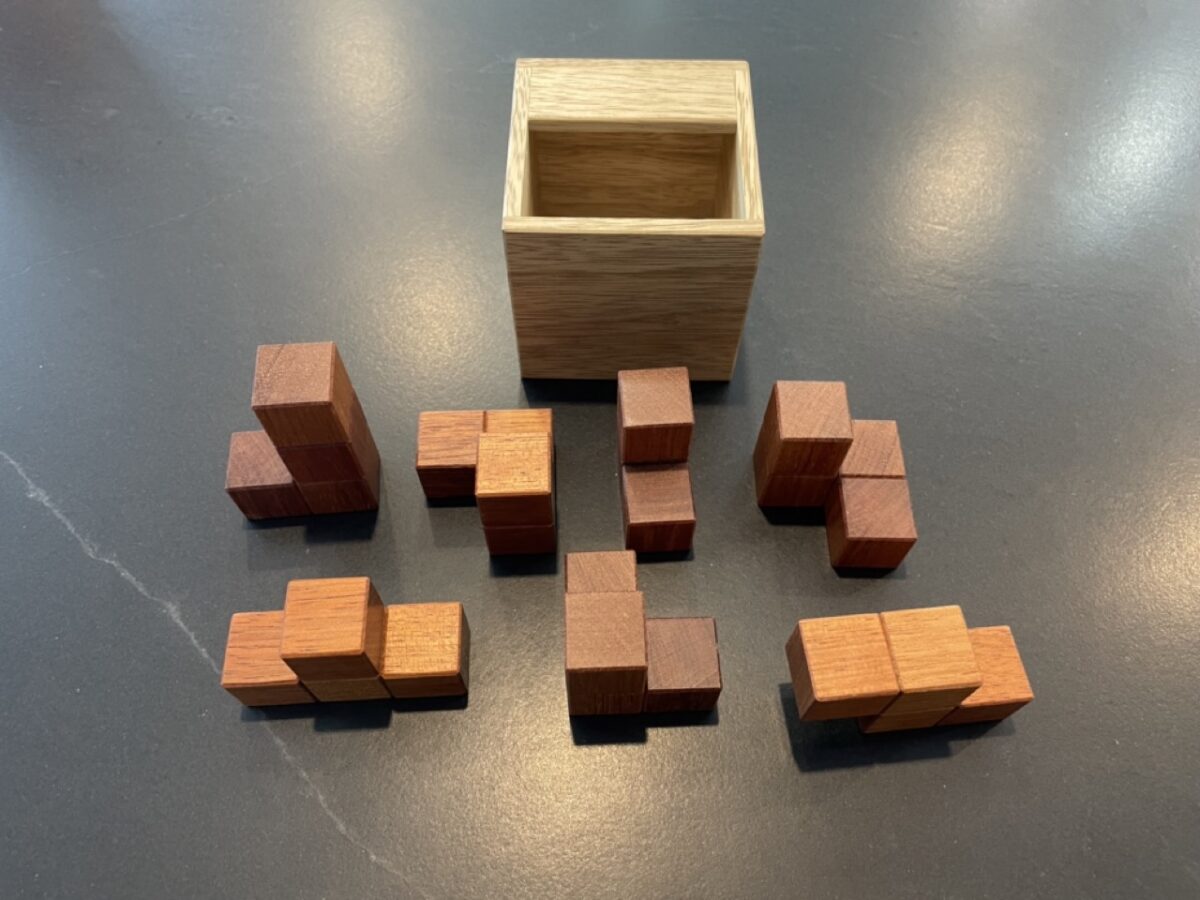
I love me some DVL puzzles. Some of the best moments I’ve had puzzling were because of the wonderful mind of Latussek. It’s hard to pin down what exactly makes his puzzles different, but I suspect it has something to do with how he uses unique shapes and restricted openings and the pieces sort of dance their way into the box.
When I saw some of the “Flop” series puzzles available at Pelikan, I had to jump on the opportunity. I purchased Soma, Fritz and Tetra Flops without knowing anything about them. Why are they all called Flop? I didn’t know at the time, but after spending some quality time with these puzzles, its become clear that they all share a similar “move” or “flop” that is part of the solution.
Today, I’m going to focus on Soma-Flop.

This was a wonderful puzzle that took me a fair bit of time to figure out, but what I like most is that it is solvable with logic and some trial and error. The solution is within reach if you remain persistent and use really think about the possibilities.
As with many packing puzzles, I started out making a cube outside the box and then tried to see if I could then fit the cube inside. This strategy, as usual, failed. There are many ways to assemble a cube and I quickly lost track of what cubes I had tried, what rotations I had tried and I found myself re-doing moves that had already failed. I needed a better strategy.
Another common approach to packing puzzles is to figure out what piece goes in last and then work from there. Using this idea, I was able to narrow it down to 3 pieces. Only 3 of the pieces could physically go in last. So, I then tried to assemble the cube using that information. It seemed to work better, but I still couldn’t find a solution.

I then really started focusing on logic and movement. If I can make a cube where piece A slides right out the top, then I know that it could be the final piece. But If A slides out, what next? Are the rest of the pieces still locked in to a cube, or can piece B then slide out and be removed? And if so, then is there a piece C that can be removed?
I eventually found an arrangement where the pieces could be removed (and thus inserted) in a sequential fashion that would work. This has to be the solution right? Well, theory and practice are 2 different things and even though the solution should work, it didn’t. There were some very stubborn pieces that I could not figure out how to maneuver into place. I backed up a few steps, but kept coming back to what I believed was the solution, but again it wouldn’t work.
And then. The magic happened. The flop revealed itself. And dammit, DVL did it again. These are such amazing moments in puzzling. I hope he knows the joy he brings to so many. Finally, I had figured it out and with some clever moves and rotations, the full cube was now inside the box. Mission accomplished.

This puzzle was just the right difficulty for me. It took a fair bit of time and reasoning, but I was able to solve it with a few hours of experimenting. Although I put it down at times and solved it over a period of days, I never felt defeated. It always seemed like the solution was just out of reach. Thus I stayed interested and avoided adding it to what Kevin Sadler calls, his “Hall of Shame” – I definitely have a embarrasing amount of puzzles in my hall of shame. But alas, this one shall instead go into my hall of triumph.